Equable Cylinder

EQUABLE CYLINDER

Balmoral Software

Solutions: 5

For a right circular cylinder with radius r and height h, we have

V = π r²h
S = 2π rh + 2π r²

By convention, the volume and the circular base area are integer multiples of π, so r²h and r² are integers and therefore h is rational. The lateral surface area is also an integer multiple of π, so 2rh is an integer and therefore r is also rational. Since r² is an integer and r is rational, it follows that r is an integer.

By equability,

r²h = 2rh + 2r²

(r - 2)(h - 2) = 4 [1]

A right cylinder is a right prism with a circular base, so h > 2 as is established elsewhere. It follows that r > 2 in any equable solution. We then have

and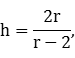

is an integer. Then r - 2 divides 16. Therefore, r ∈ {3,4,6,10,18} and we have the following five equable solutions:

r h r² 2rh V/π=S/π

3 6 9 36 54

4 4 16 32 64

6 3 36 36 108

10 5/2 100 50 250

18 9/4 324 81 729

r	h	r²	2rh	V/π=S/π
3	6	9	36	54
4	4	16	32	64
6	3	36	36	108
10	5/2	100	50	250
18	9/4	324	81	729

It follows from [1] that rh = 2(r + h), so every equable cylinder is created from a solid of rotation of an equable r x h rectangle rotated around any of its sides (although it may not have purely-integer dimensions). Three of the five equable cylinders have integer dimensions.

Top Page

Home