Balmoral Software
Solutions: 1
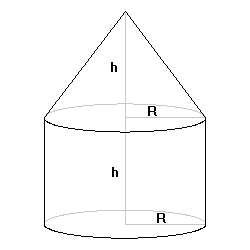
Consider the "cylindrical cone" consisting of a cone of radius R and height h surmounting a cylinder of the same radius and height. There is one such polytope that is equable. The total volume of the cone + cylinder is V, where
V/π = (1/3)R2h + R2h = (4/3)R2hThe lateral surface area of the cone is Scone, where
The lateral surface area of the cylinder is Scyl, where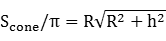
The surface area of the cylinder base is Sbase, where
Scyl/π = 2Rh [1]
The above four quantities are positive integers by convention. We have
Sbase/π = R2 [2]
so R is rational. By [2], R2 is an integer, so it follows that R is an integer.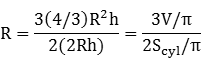
The equability assumption is
Any equable solution will have a positive left side. Squaring both sides, we have
If R = 1, then h = 12/5 and Scyl/π = 24/5 is not an integer. If R = 2, then h < 0. Therefore, R ≥ 3 and
(16R2 - 48R + 27)h = 24R2 - 36R [3]
so both the right side and the coefficient of h in [3] are strictly positive, and a rational solution for h is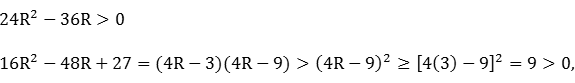
From [1],
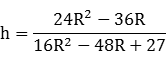
[4]
so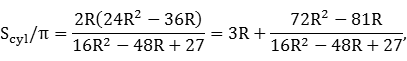
for some integer k. We previously established that the denominator is positive, and the numerator is also positive since R ≥ 3, so k is positive. The preceding equation can be written as a quadratic in R:
Since R is an integer, the discriminant of this quadratic must be a square:
(16k - 72)R2 - (48k - 81)R + 27k = 0 [5]
576k2 + 6561 = m2 for some integer mThe possibilities are summarized below:(m - 24k)(m + 24k) = 6561
The only solution is k = 15, and thus [5] can be written
m - 24k < m + 24k Sum = 2m m k = [(m + 24k) - m]/24 1 < 6561 6562 3281 (6561 - 3281)/24 is not an integer 3 < 2187 2190 1095 (2187 - 1095)/24 is not an integer 9 < 729 738 369 (729 - 369)/24 = 15 27 < 243 270 135 (243 - 135)/24 is not an integer
168R2 - 639R + 405 = 0,which has the sole integer solution R = 3. From [4], we have h = 4 and
V = 48 πThe equable cylindrical cone is a solid of revolution of a right trapezoid with sides 3, 4, 5, 8 and diagonal 5, rotated around its longest side and having perimeter 20 and area 18.Scone = 15 π
Scyl = 24 π
Sbase = 9 π
Scone2 = Sbase2 + (Scyl/2)2Therefore, any Pythagorean triple a2 + b2 = c2, for which
generates the non-equable solution
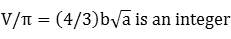
[6]
For any Pythagorean triple, an alternate solution with a and b exchanged is possible as long as [6] is satisfied. Applying the limits on a and b, we have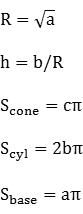
so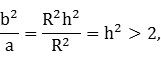
h2 > 9/16 + 81/624 ≈ 0.6923Any element of a Pythagorean triple is at least 3, so R2 = a ≥ 3 and
which reduces to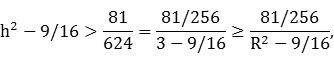
V > SconeTherefore, all solutions for which Scone ≤ M for some maximum M will include as a subset all solutions with V ≤ M. The 23 solutions having volume less than 2000 π are listed below:
R h Scone/π Sbase/π Scyl/π V/π 2 3/2 5 4 6 8 3 4 15 9 24 48 * 4 3 20 16 24 64 6 5/2 39 36 30 120 3 40/3 41 9 80 160 4 15/2 34 16 60 160 6 9/2 45 36 54 216 12 17/12 145 144 34 272 4 63/4 65 16 126 336 6 8 60 36 96 384 5 12 65 25 120 400 8 6 80 64 96 512 6 77/6 85 36 154 616 12 7/2 150 144 84 672 6 35/2 111 36 210 840 12 5 156 144 120 960 10 15/2 125 100 150 1000 6 80/3 164 36 320 1280 8 15 136 64 240 1280 9 12 135 81 216 1296 10 21/2 145 100 210 1400 7 24 175 49 336 1568 12 9 180 144 216 1728
*: Equable
Copyright © 2019 Balmoral Software (http://www.balmoralsoftware.com). All rights reserved.